Geometry has too many words: Practice with triangles

I know I mentioned this in class, but I'll say it again: Geometry has too many dang words. How am I, much less my students, supposed to remember all the terms? 

isoceles. scalene. rhombus. humanoid*. polygon.

* just making sure you're paying attention

I'll look up lots of games and tips and tricks as my career progresses, I'm sure. But meanwhile, I need to make sure I have a rock-solid understanding of the terms themselves--beginning with that basic geometric shape: the triangle.

You will NOT break me.

Let's start with the angles themselves.

A right angle is an angle at 90 degrees. We mark it with a little square, like this:

That's intuitive to me. I don't even need to think about it. So that's one down.Next:

An acute angle is less than 90 degrees . . .

. . . and an obtuse angle is greater than 90 degrees (but less than 180 degrees--duh, since 180 degrees would be a a straight line):

 Again, these terms make intuitive sense to me:

• Acute sounds like a sharp word: "Acute!"And skinny angles look sharper to me. Plus, we use the word acute to mean "sharp," as in "acute pain."

•  Obtuse sounds like a big, slow word: "Obtuuuuuse." And obtuse angles are big. Plus, we use the word obtuse to mean "blunted," or the opposite of sharp, as in, "because he hadn't read the piece, his analysis was somewhat obtuse." 

So what's the problem, then? Looks like I have these terms down, amiright? Well, hold the phone:

A

B

C

D

E

 
 Are you confused yet? I am.

I know an acute triangle has all acute angles and that an obtuse triangle has one obtuse angle. Just by looking at these five triangles, the only one that's obvious to me is E: It's an obtuse triangle because the bottom left angle clearly measures greater than 90 degrees:

 

E

I would guess that triangle A is also obtuse, because I can see clearly that the bottom right angle is wider than 90 degrees:

A

 

Triangle A is a good segue into what confuses me the most about angles/triangles, though: Which way do we measure them? 

What on earth are you talking about, Miss B?

I'll show you what I mean. Here's triangle A again--but now I've rotated it:

A

Let's look at this lower left angle. Clearly it's acute, right? Because we see what 90 degrees looks like, and this angle is less than 90 degrees.

But what about this lower right angle?

A

Again, we see what 90 degrees looks like. But the y axis of the lower right angle seems like it would create an angle greater than 90 degrees to me, because it's to the left of the 90-degree y axis. And yet, the angle doesn't look obtuse. Clearly I'm missing something.

A little obtuse, are we?

 I only see one difference between the lower left angle and the lower right angle: the direction of the x axis. The lower left angle has the x axis going to the right . . . 

A

. . . and the lower right angle has the x axis going to the left: 

A

So what if the correct way to measure an angle is to rotate it until the x axis is going to the right? Let me practice a little with my good friends at--say it with me--Khan Academy, and see if my theory holds up.

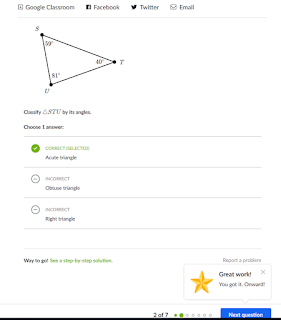
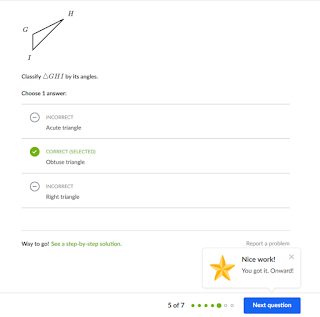
Here's a practice set for identifying triangles by angles. Here we go:

Question 1

Question 2

Question 3

Question 4

Question 5

Question 6

Question 7

 

Wahoo!

 I'm not gonna lie--I'm glad to get that achievement. But only two of those were even remotely challenging: questions 5 and 7 (the others had the angle measurements in the drawings). Still, I am delighted to report that by keeping my new rule in mind, I was able to identify those angles correctly!

And bonus: I have a trick to help my students who struggle with how to measure angles. Now, I don't know why this rule works . . . but it's good to have a little mystery in our lives:

There is more to know. . . .

 Thanks for stopping by!

 

Where have all the factors gone? Finding the LCD through factor trees

I've read your blogs. I've asked in class (a couple times, even). But I still can't figure out why.

Why do I not have to repeat all the factors to find the least common denominator (LCD)?

I know some of us have also been stumped by this question.

But for those of us who haven't, let me explain:

                               

 Let's pretend we're adding

We need to make a common denominator. We could just multiply 12 x 15 and use a denominator of 180, but that's embarrassingly huge. We could list out multiples and see what the first common one is (12, 24, 48, 60  and 15, 30, 45, 60 shows us 60 is the first one in common)--boooring. FACTOR TREE!

                                        

So here's my problem: How do I know which of these factors--2 x 2 x 3  and 3 x 5--to include in the least common denominator? Well, I could use them all, of course:

2 x 2 x 3 x 3 x 5

Which gives me what? 180. That's a common denominator, sure, because all we're doing is multiplying all the factors together, which is the same as multiplying the numbers themselves together, which we already tried (see "embarrassingly huge," above).

We're looking for the least common denominator. What if we only use the common factors, maybe? Well, the only factor (besides 1) that 12 and 15 have in common is 3, so clearly that doesn't work.

Maybe the factors that repeat cancel each other out? 

2 x 2 x 3 x 3 x 5 = 2 x 2 x 5 = 20

20 is not a common factor of 12 and 15. So there went that idea.

I'm stumped. Which means, here at Miss B's Math Trip, that it's time to head over to Khan Academy!!

Let's take a look at this video on finding common denominators:

And just like that, there it is, starting at 1:50. Seriously. Take a look. I'll wait.

The video really does explain this concept perfectly (for me, anyway). But I'll have to learn to paraphrase it for my students anyway, so here goes:

 

Let's go back to our example of finding the LCD for 5/12 and 4/15. We recall we used factor trees to get the factors of each number:

                                       

And we know we have to find a common multiple of both 12 and 15 for our denominator:

• In order to be divisible by 12, the denominator has to have factors of 2, 2, and 3.
  

• In order to be divisible by 15, the denominator has to have the factors of 3 and 5.
  

• So as long as the denominator has a 2, a 2, a 3 (both the 12 and the 15 have a 3), and a 5 in its factors, it will be divisible by both 12 and 15, because each of the factors will be there.

Remember, we want to find the lowest common denominator. And the way to do that? Just multiply the factors we know have to be there:

2 x 2 x 3x 5 = 60.

Ssssssnap!

Just to be sure I can do this in practice, though, let me do a practice set (and yes, get that sweet Level Up!)

I did have to switch up the questions a little, since the set practices other ways of finding the LCD besides using factor trees. But I got some good practice in. For example:

Which I solved like this:

And now I have officially Leveled Up!

NICE.

Thanks for stopping by!